Optimization: Newton Raphson Method
Newton Raphson Method Algorithm in C
#include<stdio.h>#include<conio.h>
#include<math.h>
#include<stdlib.h>
float myFun(float x);
int main()
{
float e = 0.01,a,aw = 0,bw = 1,Lw,b,x1,x2,fxa,fxb,fxz,z,j=0;
int i=1,k;
float x[100],dx[100],fdx[100],fddx[100];
x[1] = 1;
dx[1] = (x[i]*1)/100;
begin:
if(j<100)
{
dx[i] = (x[i]*1)/100;
fdx[i] = ((myFun(x[i] + dx[i])) - (myFun(x[i] - dx[i])))/(2*dx[i]);
fddx[i] = ((myFun(x[i] + dx[i])) - (2*myFun(x[i])) + (myFun(x[i] - dx[i])))/(dx[i]*dx[i]);
printf("\nf'(%d) = %.4f",i,fdx[i]);
printf("\nf''(%d) = %.4f",i,fddx[i]);
k = i+1;
x[k] = (x[i] - (fdx[i] / fddx[i]));
printf("\n\nf(%d) = %.4f",k,x[k]);
dx[k] = (x[k]*1)/100;
fdx[k] = ((myFun(x[k] + dx[k])) - (myFun(x[k] - dx[k])))/(2*dx[k]);
if (fdx[k] < 0)
{
fdx[k] = ((-1)*fdx[k]);
}
if (fdx[k] > e)
{
j = j + 1;
i = i + 1;
goto begin;
}
else
{
printf("\n\nf'(%d) = %.9f is Less than termination factor e = %.3f",k,fdx[k],e);
goto end;
}
}
end:
return 0;
}
float myFun(float x) {
float y;
y = ((x*x)+(54/x));
return y; // return statement
}
Output:
 |
| Newton Raphson Method |
Popular posts from this blog
VLSI: 1-4 DEMUX (Demultiplexer) Dataflow Modelling with Testbench
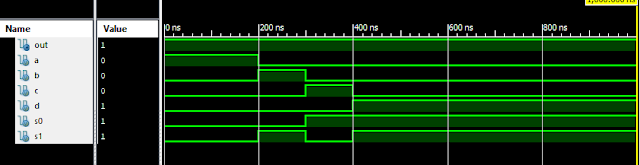
Verilog Code for 1-4 DEMUX Dataflow Modelling module demux_1_to_4( input d, input s0, input s1, output y0, output y1, output y2, output y3 ); assign s1n = ~ s1; assign s0n = ~ s0; assign y0 = d& s0n & s1n; assign y1 = d & s0 & s1n; assign y2 = d & s0n & s1; assign y3 = d & s0 & s1; endmodule //Testbench code for 1-4 DEMUX Dataflow Modelling initial begin // Initialize Inputs ...
VLSI: 4-1 MUX Dataflow Modelling with Testbench
Verilog Code for 4-1 MUX Dataflow Modelling module m41(out, i0, i1, i2, i3, s0, s1); output out; input i0, i1, i2, i3, s0, s1; assign y0 = (i0 & (~s0) & (~s1)); assign y1 = (i1 & (~s0) & s1); assign y2 = (i2 & s0 & (~s1)); assign y3 = (i3 & s0 & s1); assign out = (y0 | y1 | y2 | y3); endmodule //Testbench code for 4-1 MUX Dataflow Modelling initial begin // Initialize Inputs a = 1;b = 0;c = 0;d = 0;s0 = 0;s1 = 0; ...
Full Subtractor Verilog Code in Structural/Gate Level Modelling with Testbench
Verilog Code for Full Subtractor Structural/Gate Level Modelling module full_sub(borrow,diff,a,b,c); output borrow,diff; input a,b,c; wire w1,w4,w5,w6; xor (diff,a,b,c); not n1(w1,a); and a1(w4,w1,b); and a2(w5,w1,c); and a3(w6,b,c); or o1(borrow,w4,w5,w6); endmodule //Testbench code for Full Subtractor Structural/Gate Level Modelling initial begin // Initialize Inputs a = 0; b = 0; c = 0; // Wait 100 ns for global reset to finish #100; // Add stimulus here #100; a = 0;b = 0;c = 1; #100; a = 0;b = 1;c = 0; #100; a = 0;b = 1;c = 1; #100; a = 1;b = 0;c = 0; #100; a = 1;b = 0;c = 1; #100; a = 1;b = 1;c = 0; #100; a = 1;b = 1;c = 1; end Output: RTL Schematic: Full Subtractor Verilog Other Verilog Programs: Go to Index of Verilog Programming
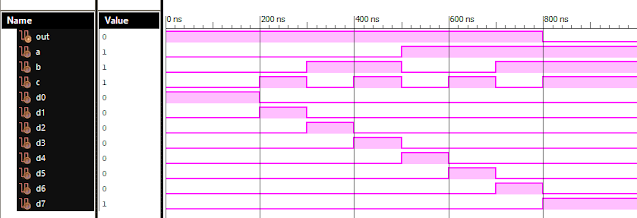
Verilog: 8 to 1 Multiplexer (8-1 MUX) Dataflow Modelling with Testbench Code
Verilog Code for 8 to 1 Multiplexer Dataflow Modelling module mux_8to1( input a, input b, input c, input D0, input D1, input D2, input D3, input D4, input D5, input D6, input D7, output out, ); module m81( output out, input D0, D1, D2, D3, D4, D5, D6, D7, S0, S1, S2); assign S1bar=~S1; assign S0bar=~S0; assign S2bar=~S2; assign out = (D0 & S2bar & S1bar & S0bar) | (D1 & S2bar & S1bar & S0) | (D2 & S2bar & S1 & S0bar) + (D3 & S2bar & S1 & S0) + (D4 & S2 & S1bar & S0bar) + (D5 & S2 & S1bar & S0) + (D6 & S2 & S1 & S0bar) + (D7 & S2 & S1 & S0); endmodule //Testbench code for 8-1 MUX Dataflow Modelling initial begin // Initialize Inputs a= 0;b = 0;c = 0;D0 = 1;D1 = 0;D2 = 0;D3 = 0;D4 = 0;D5 = 0;D6 = 0;D7 = 0; // Wait 100 ns for global reset to finish #100; // Add stimulus here #100; a = 0;b = 0;c = 1;d0 = ...
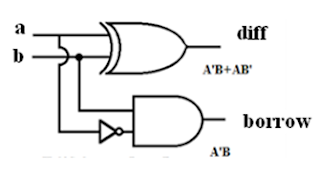
VLSI: Half Subtractor and Full Subtractor Gate Level Modelling
Half Subtractor: Verilog Module Code: module half_subtractor ( input a, input b, output diff output borr ); wire x; xor (diff,a,b); not (x,a); and (borr,x,b); endmodule Full Subtractor: Verilog Module Code: module full_subtractor ( input a, input b, input c, output diff output borr ); wire x,n2,z,n1; xor s1(x,a,b); not s3(n2,x); not s4(n1,c); and s5(y,n1,b); xor s2(diff,a,x); and s6(z,n2,a); or (borr,y,z); endmodule
Comments
Post a Comment