Latest Post

Space Facts: Part 8 - Birth of Universe, Cosmic Inflation

-
The Birth of Universe:
It is believed that before Big bang universe was a tiny point having infinite density and infinite temperature. Surrounding of that point was void, having no matter, no temperature and no time.
After Big-Bang (a great explosion from that singular point) the 'matter' spread in all directions at the speed of light. According to theory of relativity, if an object travels at a speed of light, time dimension for that object becomes zero. In other words time stops for that object. So within no time that minute zero dimensional point became an expanding universe. This expansion of Universe after Big-Bang is called Cosmic Inflation.

Now what is Cosmic Inflation Theory?

Cosmos means Universe and Inflation refers to Spread of Universe. Big-Bang theory does not explain from where that point universe came into existence and what happened after Big-Bang. Cosmic Inflation Theory explains what happened after Big-Bang.
Cosmic Inflation Theory starts when the speed of inflation decreased than speed of light. That's the reason why Big-Bang theory is explained in 3 dimensions but Cosmic Inflation Theory is explained in 4 dimensions. The 4th dimension is Time. Time started when universe was spreading with speed lower than the speed of light.

What Einstein thought on Cosmic Inflation?

Einstein compared the universe with balloon. Balloon inflates till we blow air in it and shrinks after reaching a saturation point. Using this assumption he gave his "Special Theory of Relativity".
But today most of the Astrophysicists believe that universe will not shrink and will keep on expanding. Recent discoveries also put light on theory, that universe is still expanding but an uneven expansion of Universe is taking place.
Although Einstein made some mistakes in his assumptions most of the equations given by his theory of relativity has proved to be true!!

Comments

Post a Comment

Popular posts from this blog

VLSI: 1-4 DEMUX (Demultiplexer) Dataflow Modelling with Testbench

-
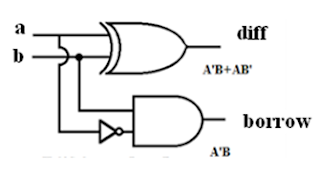
Image
Verilog Code for 1-4 DEMUX Dataflow Modelling module demux_1_to_4(     input d,     input s0,     input s1,     output y0,     output y1,     output y2,     output y3     ); assign s1n = ~ s1; assign s0n = ~ s0; assign y0 = d& s0n & s1n; assign y1 = d & s0 & s1n; assign y2 = d & s0n & s1; assign y3 = d & s0 & s1; endmodule //Testbench code for 1-4 DEMUX Dataflow Modelling initial begin                              // Initialize Inputs ...
Read more

Full Subtractor Verilog Code in Structural/Gate Level Modelling with Testbench

-
Image
Verilog Code for Full Subtractor Structural/Gate Level Modelling module  full_sub(borrow,diff,a,b,c); output  borrow,diff; input  a,b,c; wire w1,w4,w5,w6; xor (diff,a,b,c); not n1(w1,a); and a1(w4,w1,b); and a2(w5,w1,c); and a3(w6,b,c); or o1(borrow,w4,w5,w6); endmodule //Testbench code for Full Subtractor Structural/Gate Level Modelling initial  begin // Initialize Inputs a = 0; b = 0; c = 0; // Wait 100 ns for global reset to finish #100; // Add stimulus here #100; a = 0;b = 0;c = 1; #100; a = 0;b = 1;c = 0; #100; a = 0;b = 1;c = 1; #100; a = 1;b = 0;c = 0; #100; a = 1;b = 0;c = 1; #100; a = 1;b = 1;c = 0; #100; a = 1;b = 1;c = 1; end Output: RTL Schematic: Full Subtractor Verilog Other Verilog Programs: Go to Index of  Verilog Programming
Read more

VLSI: Half Subtractor and Full Subtractor Gate Level Modelling

-
Image
Half Subtractor: Verilog Module Code: module half_subtractor (      input  a,      input  b,      output  diff       output  borr ); wire x; xor (diff,a,b); not (x,a); and (borr,x,b); endmodule Full Subtractor: Verilog Module Code: module full_subtractor (      input  a,      input  b,      input  c,      output  diff       output  borr ); wire x,n2,z,n1; xor s1(x,a,b); not s3(n2,x); not s4(n1,c); and s5(y,n1,b); xor s2(diff,a,x); and s6(z,n2,a); or (borr,y,z); endmodule
Read more

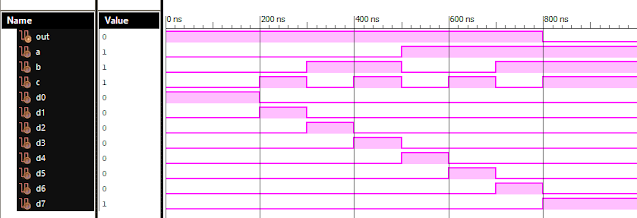
Verilog: 8 to 1 Multiplexer (8-1 MUX) Dataflow Modelling with Testbench Code

-
Image
  Verilog Code for 8 to 1 Multiplexer Dataflow Modelling module mux_8to1(     input a, input b, input c, input D0, input D1, input D2, input D3, input D4, input D5, input D6, input D7, output out, ); module m81( output out, input D0, D1, D2, D3, D4, D5, D6, D7, S0, S1, S2); assign S1bar=~S1; assign S0bar=~S0; assign S2bar=~S2; assign out = (D0 & S2bar & S1bar & S0bar) | (D1 & S2bar & S1bar & S0) | (D2 & S2bar & S1 & S0bar) + (D3 & S2bar & S1 & S0) + (D4 & S2 & S1bar & S0bar) + (D5 & S2 & S1bar & S0) + (D6 & S2 & S1 & S0bar) + (D7 & S2 & S1 & S0); endmodule //Testbench code for 8-1 MUX Dataflow Modelling initial begin // Initialize Inputs a= 0;b = 0;c = 0;D0 = 1;D1 = 0;D2 = 0;D3 = 0;D4 = 0;D5 = 0;D6 = 0;D7 = 0; // Wait 100 ns for global reset to finish #100; // Add stimulus here #100; a = 0;b = 0;c = 1;d0 = ...
Read more

VLSI: 8-3 Encoder Dataflow Modelling with Testbench

-
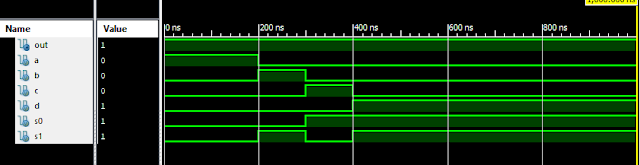
Image
Verilog Code for 8-3 Encoder Dataflow Modelling module encoder_8_to_3(     input d0,     input d1,     input d2,     input d3,     input d4,     input d5,     input d6,     input d7,     output q0,     output q1,     output q2     ); assign q0 = ( d1 | d3 | d5 | d7 ); assign q1 = ( d2 | d3 | d6 | d7 ); assign q2 = ( d4 | d6 | d5 | d7 ); endmodule //Testbench code for 8-3 Encoder Dataflow Modelling initial begin                         ...
Read more